Effect of Sloping Surcharge in Active Case | Bestengineeringprojects.com

What are the Effect of Sloping Surcharge in Active Case?

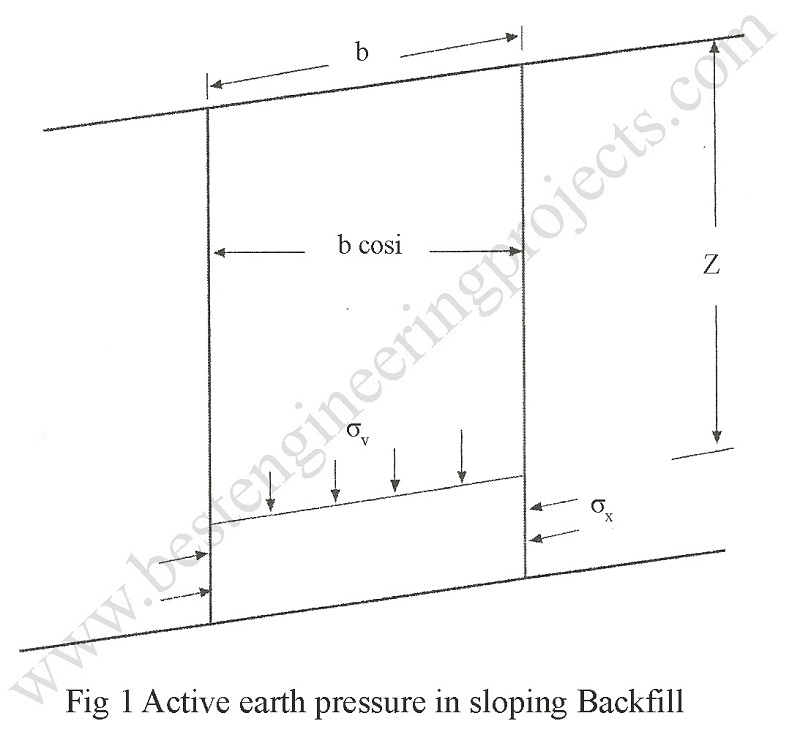
in this article we will discuss about Effect of Sloping Surcharge in Active Case. A wall carrying a sloping backfill is shown in Fig.1. Consider an element subjected to a stress $\sigma_v$ in the vertical direction and $\sigma_x$ in the direction parallel to the backfill. As these stresses in one plane are parallel to the direction of another plane, these stresses are conjugate stresses and the planes are the conjugate planes. The equivalent vertical stress acting parallel to the surface of backfill is given by:

$\sigma_{ve} = \sigma_v cosi$ ———- (1)

$\sigma_{ve} = \gamma z cosi$

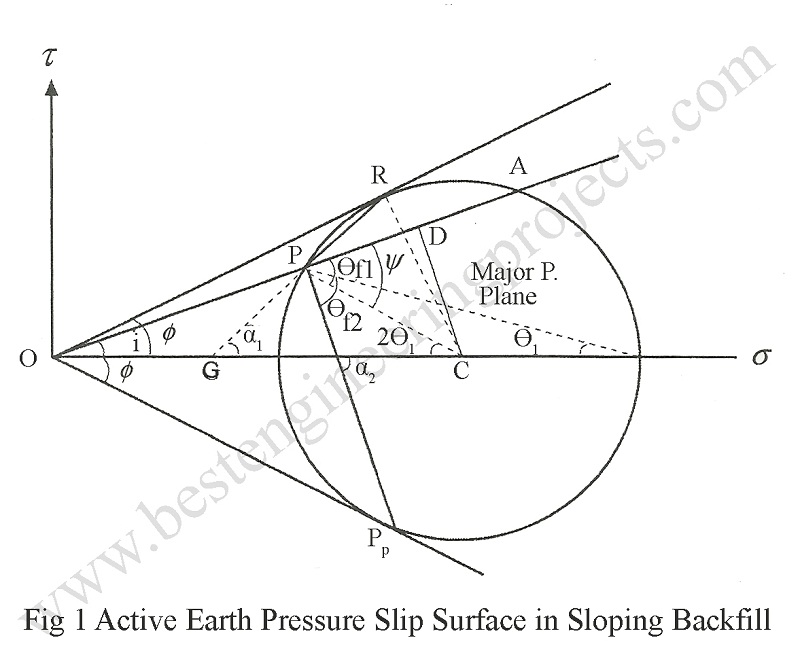
The Mohr Circle, which represents this case of inclined backfill is shown in the Fig 1.

Referring to the figure above (figure 1), the coefficient of active earth pressure can be written as:

$K_a=\dfrac{\sigma_x}{\sigma_{ve}}=\dfrac{P_a}{yz cosi}$

$=\dfrac{OB}{OA}=\dfrac{OP}{OA}$

$=\dfrac{OD-PD}{OD+AD}$

$=\dfrac{OD-PD}{OD+PD}$

We can also write:

CD = OC sini

OD = OC cosi

$PD=\sqrt{PC^2-CD^2}$

$PD=\sqrt{R^2-CD^2}$ Because PC = R

$= OC sin\phi$

By substitution these values in the expression for K_a we get;

$K_a=\dfrac{OCcosi-\sqrt{OC^2sin^2\phi-OC^2sin^2i}}{OCcosi+\sqrt{OC^2sin^2\phi-OC^2sin^2i}}$

$K_a=\dfrac{cosi-\sqrt{sin^2\phi-sin^2i}}{cosi+\sqrt{sin^2\phi-sin^2i}}=\dfrac{cosi-\sqrt{cos^2i-cos^2\phi}}{cosi+\sqrt{cos^2i-cos^2\phi}}$

Therefore, $P_a = K_a\gamma z cosi = K_A\gamma z$

Where,

$K_A = K_acosi = K_acosi[\dfrac{cosi-\sqrt{cos^2i-cos^2\phi}}{cosi+\sqrt{cos^2i-cos^2\phi}}]$ and is known as the coefficient of active earth pressure.